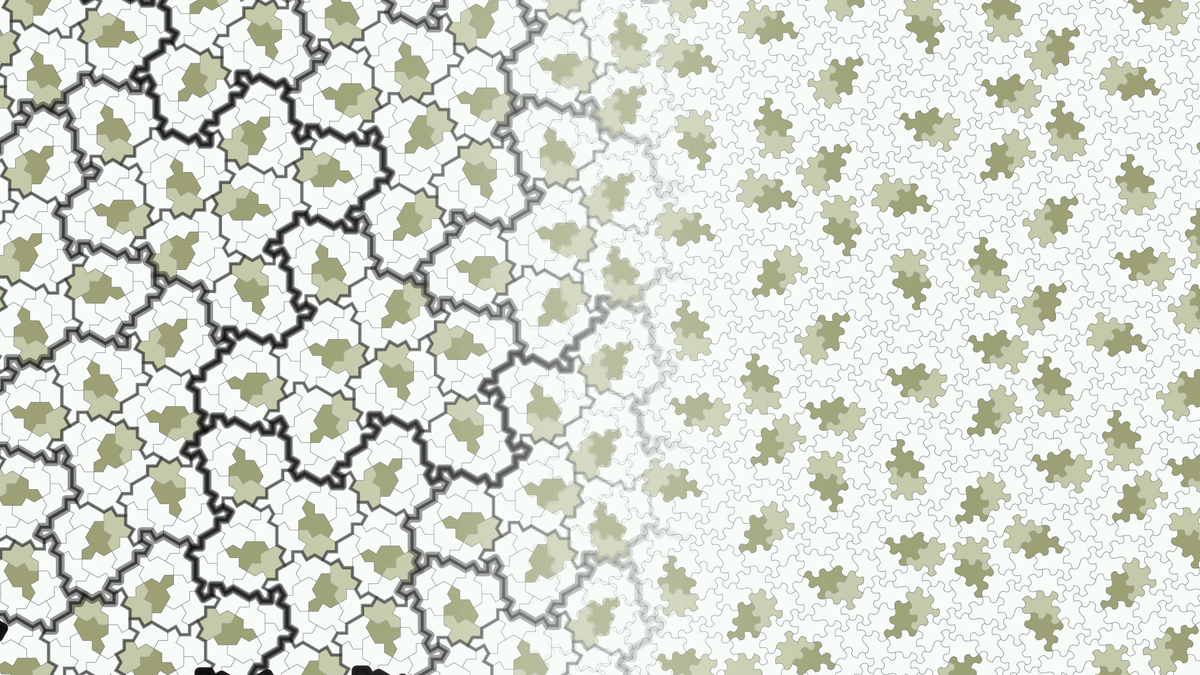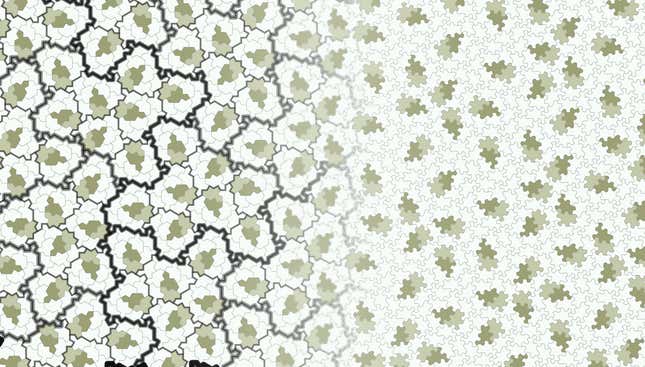
In March, a group of mathematicians identified a thirteen-sided shape called “the hat” that can tile a surface without any patterns repeating. Now the researchers are updating the shape to maintain its quirky tiling properties while addressing an important caveat.
The new type of shape is called a “spectre” and it’s what’s known as an aperiodic monotile, which means that a single shape can tile a surface without any translational symmetry, or without its pattern ever repeating. The famous Penrose tilings are an example of aperiodic tiling, in which the pattern is aperiodic but uses two different shapes. The hat tiling discovered by the researchers just a few months ago technically uses one shape, except that in order to tile a surface, you’d need to use both the hat and its mirrored reflection—meaning you’d technically need two shapes. The spectre is described in a new preprint paper from David Smith and his colleagues.
“In plane tiling, it is completely standard that tiles may be reflected; nevertheless, some people were dissatisfied that the aperiodic hat monotile requires reflections to tile the plane,” co-author Joseph Samuel Meyers wrote on Mastodon. “In our new preprint, we present the Spectre, the first example of a vampire einstein: an aperiodic monotile that tiles the plane without reflections.”
The spectre tiling only uses one shape, an “einstein,” which is German for “one stone,” to completely tile a surface without any part of the pattern repeating. Since the spectre doesn’t need to be reflected like the hat does, the researchers dubbed the shape as a “vampire einstein”—a reference to how the humanoid bloodsuckers don’t show up in mirrors.
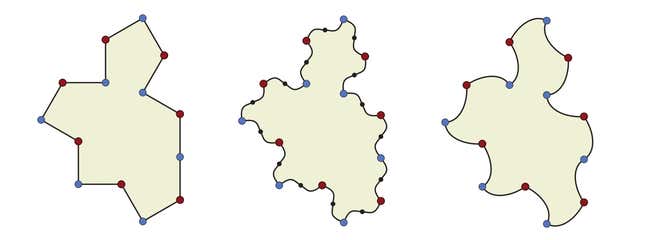
The hat is a 13-sided polykite shape consisting of eight kites connected at their edges, and is referred to mathematically as a “weakly chiral aperiodic monotile,” in which both the tile and its reflection are needed to tile a surface without repeating. Smith and his collaborators found a 14-sided shape with straight edges that also needs its reflection to tile a surface without repeating any patterns. However, after curving each sides of the 14-sided shape, the researchers found that the spectre alone could tile a surface without repeating and refer to this as a “strictly chiral aperiodic monotile.”
For most of us, the presence of the spectre is nothing to sneeze at, but for the mathematics community, it answers a longstanding, hypothetical conundrum in geometry.